-
ĐẠI SỐ VÀ GIẢI TÍCH - TOÁN 11
-
HÌNH HỌC - TOÁN 11
-
CHƯƠNG I. PHÉP DỜI HÌNH VÀ PHÉP ĐỒNG DẠNG TRONG MẶT PHẲNG
-
CHƯƠNG II. ĐƯỜNG THẲNG VÀ MẶT PHẲNG TRONG KHÔNG GIAN. QUAN HỆ SONG SONG
- Bài 1. Đại cương về đường thẳng và mặt phẳng
- Bài 2. Hai đường thẳng chéo nhau và hai đường thẳng song song
- Bài 3. Đường thẳng và mặt phẳng song song
- Bài 4. Hai mặt phẳng song song
- Bài 5. Phép chiếu song song. Hình biểu diễn của một hình không gian
- Ôn tập chương II - Đường thẳng và mặt phẳng trong không gian. Quan hệ song song
-
CHƯƠNG III. VECTƠ TRONG KHÔNG GIAN. QUAN HỆ VUÔNG GÓC TRONG KHÔNG GIAN
-
ÔN TẬP CUỐI NĂM HÌNH HỌC - TOÁN 11
-
-
Câu hỏi tự luyện Toán 11
-
TẢI 50 ĐỀ KIỂM TRA 15 PHÚT TOÁN 11
-
TẢI 30 ĐỀ KIỂM TRA 1 TIẾT TOÁN 11
-
TẢI 5 ĐỀ THI GIỮA KÌ 1 TOÁN 11
-
TẢI 25 ĐỀ THI HỌC KÌ 1 TOÁN 11
-
TẢI 6 ĐỀ THI GIỮA HỌC KÌ 2 TOÁN 11
-
TẢI 20 ĐỀ THI HỌC KÌ 2 TOÁN 11
Giải toán 11, giải bài tập toán lớp 11 đầy đủ đại số và giải tích, hình học
Bài 1. Vectơ trong không gian
Bài 8 trang 92 SGK Hình học 11
Cho hình lăng trụ tam giác ABC.A'B'C' có...
Đề bài
Cho hình lăng trụ tam giác \(ABC.A'B'C'\) có \(\overrightarrow{AA'}\) = \(\overrightarrow{a}\), \(\overrightarrow{AB}\) = \(\overrightarrow{b}\), \(\overrightarrow{AC}\) = \(\overrightarrow{c}\). Hãy phân tích (hay biểu thị véctơ \(\overrightarrow{B'C}\), \(\overrightarrow{BC'}\) qua các véctơ \(\overrightarrow{a}\),\(\overrightarrow{b}\), \(\overrightarrow{c}\).
Video hướng dẫn giải
Phương pháp giải - Xem chi tiết
Xen điểm thích hợp để làm xuất hiện các véc tơ \(\overrightarrow{a}\),\(\overrightarrow{b}\), \(\overrightarrow{c}\), sử dụng các cặp vecto bằng nhau và bằng các vecto đã cho.
Lời giải chi tiết
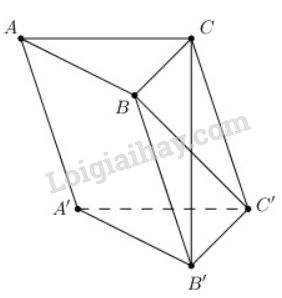
\(\eqalign{& \overrightarrow {B'C} = \overrightarrow {B'A'} + \overrightarrow {A'A} + \overrightarrow {AC} \cr &= - \overrightarrow {AB} - \overrightarrow {AA'} + \overrightarrow {AC} \cr &= - \overrightarrow b - \overrightarrow a + \overrightarrow c \cr & \overrightarrow {BC'} = \overrightarrow {BA} + \overrightarrow {AA'} + \overrightarrow {A'C'} \cr & = - \overrightarrow {AB} + \overrightarrow {AA'} + \overrightarrow {AC} \cr &= - \overrightarrow b + \overrightarrow a + \overrightarrow c \cr} \)
Nhận xét: Ba véctơ \(\overrightarrow{a}; \overrightarrow{b}; \overrightarrow{c}\) ở trên gọi là bộ ba véctơ cơ sở (dùng để phân tích các véctơ khác).
Cách khác:
\(\begin{array}{l}
\overrightarrow {B'C} = \overrightarrow {AC} - \overrightarrow {AB'} \\
= \overrightarrow {AC} - \left( {\overrightarrow {AB} + \overrightarrow {BB'} } \right)\\
= \overrightarrow {AC} - \overrightarrow {AB} - \overrightarrow {BB'} \\
= \overrightarrow {AC} - \overrightarrow {AB} - \overrightarrow {AA'} \\
= \overrightarrow c - \overrightarrow b - \overrightarrow a \\
\overrightarrow {BC'} = \overrightarrow {AC'} - \overrightarrow {AB} \\
= \overrightarrow {AA'} + \overrightarrow {A'C'} - \overrightarrow {AB} \\
= \overrightarrow {AA'} + \overrightarrow {AC} - \overrightarrow {AB} \\
= \overrightarrow a + \overrightarrow c - \overrightarrow b
\end{array}\)
Loigiaihay.com
Bình luận
Chia sẻ
- Bài 9 trang 92 SGK Hình học 11
- Bài 10 trang 92 SGK Hình học 11
- Bài 7 trang 92 SGK Hình học 11
- Bài 6 trang 92 SGK Hình học 11
- Bài 5 trang 92 SGK Hình học 11
>> Xem thêm
Luyện Bài Tập Trắc nghiệm Toán 11 - Xem ngay
Tham Gia Group Dành Cho 2K8 Chia Sẻ, Trao Đổi Tài Liệu Miễn Phí
