-
ĐẠI SỐ VÀ GIẢI TÍCH - TOÁN 11
-
HÌNH HỌC - TOÁN 11
-
CHƯƠNG I. PHÉP DỜI HÌNH VÀ PHÉP ĐỒNG DẠNG TRONG MẶT PHẲNG
-
CHƯƠNG II. ĐƯỜNG THẲNG VÀ MẶT PHẲNG TRONG KHÔNG GIAN. QUAN HỆ SONG SONG
- Bài 1. Đại cương về đường thẳng và mặt phẳng
- Bài 2. Hai đường thẳng chéo nhau và hai đường thẳng song song
- Bài 3. Đường thẳng và mặt phẳng song song
- Bài 4. Hai mặt phẳng song song
- Bài 5. Phép chiếu song song. Hình biểu diễn của một hình không gian
- Ôn tập chương II - Đường thẳng và mặt phẳng trong không gian. Quan hệ song song
-
CHƯƠNG III. VECTƠ TRONG KHÔNG GIAN. QUAN HỆ VUÔNG GÓC TRONG KHÔNG GIAN
-
ÔN TẬP CUỐI NĂM HÌNH HỌC - TOÁN 11
-
-
Câu hỏi tự luyện Toán 11
-
TẢI 50 ĐỀ KIỂM TRA 15 PHÚT TOÁN 11
-
TẢI 30 ĐỀ KIỂM TRA 1 TIẾT TOÁN 11
-
TẢI 5 ĐỀ THI GIỮA KÌ 1 TOÁN 11
-
TẢI 25 ĐỀ THI HỌC KÌ 1 TOÁN 11
-
TẢI 6 ĐỀ THI GIỮA HỌC KÌ 2 TOÁN 11
-
TẢI 20 ĐỀ THI HỌC KÌ 2 TOÁN 11
Giải toán 11, giải bài tập toán lớp 11 đầy đủ đại số và giải tích, hình học
Bài 2. Hai đường thẳng vuông góc
Bài 8 trang 98 SGK Hình học 11
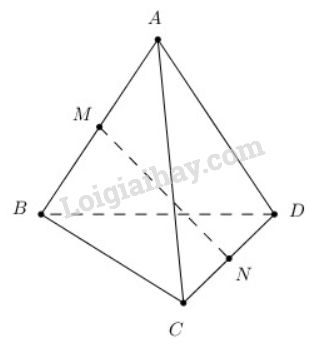
Cho tứ diện ABCD có AB = AC = AD ...
Đề bài
Cho tứ diện \(ABCD\) có \(AB = AC = AD\) và \(\widehat{BAC}=\widehat{BAD}=60^{0}.\) Chứng minh rằng:
a) \(AB ⊥ CD\);
b) Nếu \(M, N\) lần lượt là trung điểm của \(AB\) và \(CD\) thì \(MN ⊥ AB\) và \(MN ⊥ CD\).
Video hướng dẫn giải
Lời giải chi tiết
a) \(\overrightarrow{AB}.\overrightarrow{CD}=\overrightarrow{AB}(\overrightarrow{AD}-\overrightarrow{AC})\)
\(=\overrightarrow{AB}.\overrightarrow{AD}-\overrightarrow{AB}.\overrightarrow{AC}\)
\(=AB.AD.\cos\widehat{BAD}-AB.AC.\cos\widehat{BAC} =0\)
Cộng (1) với (2) theo vế với vế ta được:
\(\begin{array}{l}
2\overrightarrow {MN} \\
= \left( {\overrightarrow {MA} + \overrightarrow {MB} } \right) + \left( {\overrightarrow {AD} + \overrightarrow {BC} } \right) + \left( {\overrightarrow {DN} + \overrightarrow {CN} } \right)\\
= \overrightarrow 0 + \left( {\overrightarrow {AD} + \overrightarrow {BC} } \right) + \overrightarrow 0 \\
= \overrightarrow {AD} + \overrightarrow {BC} \\
\Rightarrow \overrightarrow {MN} = \dfrac{1}{2}\left( {\overrightarrow {AD} + \overrightarrow {BC} } \right)\\
= \dfrac{1}{2}\left( {\overrightarrow {AD} + \overrightarrow {AC} - \overrightarrow {AB} } \right)
\end{array}\)
Ta có \(\overrightarrow{AB}.\overrightarrow{MN}={1 \over 2}\overrightarrow {AB} .(\overrightarrow {AD} + \overrightarrow {AC} - \overrightarrow {AB} )\)
\(= {1 \over 2}(\overrightarrow {AB} .\overrightarrow {AD} + \overrightarrow {AB} .\overrightarrow {AC} - A{B^2})\)
\(= {1 \over 2}(AB.AD.\cos\widehat{BAD}+AB.AC.\cos\widehat{BAC}-AB^2)\)
\(={1 \over 2}(AB.AD.\cos60^0+AB.AC.\cos60^0-AB^2)\)
\(={1 \over 2}\left({1 \over 2}AB^2+{1 \over 2}AB^2-AB^2\right)=0\) \(\Rightarrow AB ⊥ MN\).
\(\begin{array}{l}
\overrightarrow {MN} .\overrightarrow {CD} \\
= \dfrac{1}{2}\left( {\overrightarrow {AD} + \overrightarrow {AC} - \overrightarrow {AB} } \right).\left( {\overrightarrow {AD} - \overrightarrow {AC} } \right)\\
= \dfrac{1}{2}\left( {{{\overrightarrow {AD} }^2} + \overrightarrow {AC} .\overrightarrow {AD} - \overrightarrow {AB} .\overrightarrow {AD} - \overrightarrow {AC} .\overrightarrow {AD} - {{\overrightarrow {AC} }^2} + \overrightarrow {AB} .\overrightarrow {AC} } \right)\\
= \dfrac{1}{2}\left( {A{D^2} - \overrightarrow {AB} .\overrightarrow {AD} - A{C^2} + \overrightarrow {AB} .\overrightarrow {AC} } \right)\\
= \dfrac{1}{2}\left( {A{D^2} - AB.AC\cos \widehat {BAD} - A{C^2} + AB.AC.\cos \widehat {BAC}} \right)\\
= \dfrac{1}{2}\left( {A{B^2} - A{B^2}\cos {{60}^0} - A{B^2} + A{B^2}\cos {{60}^0}} \right)\\
= \dfrac{1}{2}.0 = 0\\
\Rightarrow MN \bot CD
\end{array}\)
Loigiaihay.com
Bình luận
Chia sẻ
- Bài 7 trang 98 SGK Hình học 11
- Bài 6 trang 98 SGK Hình học 11
- Bài 5 trang 98 SGK Hình học 11
- Bài 4 trang 98 SGK Hình học 11
- Bài 3 trang 97 SGK Hình học 11
>> Xem thêm
Luyện Bài Tập Trắc nghiệm Toán 11 - Xem ngay
Tham Gia Group Dành Cho 2K8 Chia Sẻ, Trao Đổi Tài Liệu Miễn Phí
