Giải SBT toán hình học và đại số giải tích 11 cơ bản Bài 2: Hai đường thẳng vuông góc

Bài 3.9 trang 138 SBT hình học 11


Giải bài 3.9 trang 138 sách bài tập hình học 11. Cho tứ giác ABCD. Gọi M, N, P, Q lần lượt là trung điểm của các đoạn AC, BD, AD và có MN = PQ . Chứng minh rằng AB ⊥ CD...

Đề bài

Cho tứ giác \(ABCD\). Gọi \(M, N, P, Q\) lần lượt là trung điểm của các đoạn \(AC, BD, AD\) và có \(MN = PQ\). Chứng minh rằng \(AB ⊥ CD\).

Phương pháp giải - Xem chi tiết

Ta cần chứng minh \(\overrightarrow {AB} .\overrightarrow {C{\rm{D}}}  = 0\)

Quảng cáo

Lộ trình SUN 2025

Lời giải chi tiết

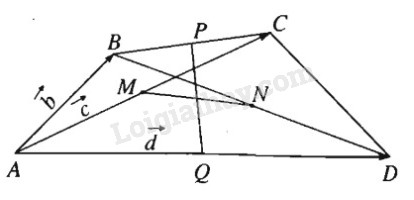
Ta cần chứng minh \(\displaystyle \overrightarrow {AB} .\overrightarrow {C{\rm{D}}}  = 0\)

Đặt \(\displaystyle \overrightarrow {AB}  = \overrightarrow b ,\,\,\overrightarrow {AC}  = \overrightarrow c ,\,\,\overrightarrow {AD}  = \overrightarrow d \). Ta có:

\(\displaystyle \overrightarrow {MN}  = \overrightarrow {MA}  + \overrightarrow {AN}\) \(\displaystyle   =  - {1 \over 2}\overrightarrow {AC}  + {1 \over 2}\left( {\overrightarrow {AB}  + \overrightarrow {AD} } \right)\)

Suy ra \(\displaystyle \overrightarrow {MN}  = {1 \over 2}\left( {\overrightarrow b  + \overrightarrow d  - \overrightarrow c } \right)\)

\(\displaystyle \eqalign{
& \overrightarrow {QP} = \overrightarrow {QA} + \overrightarrow {AP} \cr 
& = - {1 \over 2}\overrightarrow {A{\rm{D}}} + {1 \over 2}\left( {\overrightarrow {AB} + \overrightarrow {AC} } \right) \cr 
& = {1 \over 2}\left( {\overrightarrow b + \overrightarrow c - \overrightarrow d } \right) \cr} \)

Theo giả thiết ta có:

\(\displaystyle MN = PQ \Leftrightarrow {\overrightarrow {MN} ^2} = {\overrightarrow {QP} ^2}\)

\(\displaystyle \eqalign{
& {\left( {\overrightarrow b + \overrightarrow d - \overrightarrow c } \right)^2} = {\left( {\overrightarrow b + \overrightarrow c - \overrightarrow d } \right)^2} \cr 
& \Leftrightarrow \overrightarrow b .\overrightarrow d - \overrightarrow b .\overrightarrow c = \overrightarrow b .\overrightarrow c - \overrightarrow b .\overrightarrow d \cr 
& \Leftrightarrow 2\overrightarrow b .\overrightarrow d - 2\overrightarrow b .\overrightarrow c = 0 \cr 
& \Leftrightarrow \overrightarrow b .\left( {\overrightarrow d - \overrightarrow c } \right) = 0 \cr 
& \Leftrightarrow \overrightarrow {AB} .\left( {\overrightarrow {A{\rm{D}}} - \overrightarrow {AC} } \right) = 0 \cr 
& \Leftrightarrow \overrightarrow {AB} .\overrightarrow {C{\rm{D}}} = 0 \Leftrightarrow \overrightarrow {AB} \bot \overrightarrow {C{\rm{D}}} \cr} \)

 Loigiaihay.com


Bình chọn:
4.9 trên 7 phiếu
Bài tiếp theo

>> Xem thêm

Luyện Bài Tập Trắc nghiệm Toán 11 - Xem ngay

Báo lỗi - Góp ý

2K7 tham gia ngay group để nhận thông tin thi cử, tài liệu miễn phí, trao đổi học tập nhé!

>> Lộ Trình Sun 2025 - 3IN1 - 1 lộ trình ôn 3 kì thi (Luyện thi TN THPT & ĐGNL; ĐGTD) tại Tuyensinh247.com. Đầy đủ theo 3 đầu sách, Thầy Cô giáo giỏi, 3 bước chi tiết: Nền tảng lớp 12; Luyện thi chuyên sâu; Luyện đề đủ dạng đáp ứng mọi kì thi.

Các bài khác cùng chuyên mục


App Loigiaihay trên google play store App Loigiaihay trên apple store

Copyright © 2021 loigiaihay.com

DMCA.com Protection Status
App Loigiaihay trên apple store App Loigiaihay trên google play store

Đăng ký để nhận lời giải hay và tài liệu miễn phí

Cho phép loigiaihay.com gửi các thông báo đến bạn để nhận được các lời giải hay cũng như tài liệu miễn phí.

Đồng ý Bỏ qua