Bài 1. Tọa độ của vecto Toán 10 Cánh diều

Giải bài 1 trang 65 SGK Toán 10 tập 2 – Cánh diều

Tìm tọa độ của các vecto trong Hình 16 và biểu diễn mỗi vecto đó qua hai vecto

Tổng hợp đề thi học kì 1 lớp 10 tất cả các môn - Cánh diều

Toán - Văn - Anh - Lí - Hóa - Sinh - Sử - Địa...

Đề bài

Tìm tọa độ của các vecto trong Hình 16 và biểu diễn mỗi vecto đó qua hai vecto \(\overrightarrow i , \overrightarrow j \)

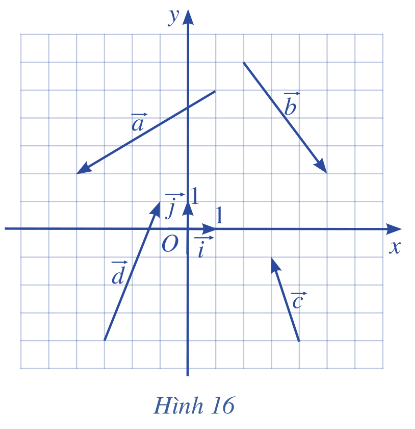
Phương pháp giải - Xem chi tiết

Vẽ các vecto \(\overrightarrow {OA} = \overrightarrow a ,\overrightarrow {OB} = \overrightarrow b ,\overrightarrow {OC} = \overrightarrow c ,\overrightarrow {OD} = \overrightarrow d \). Tọa độ của 4 điểm A, B, C, D là tọa độ của 4 vecto.

Để biểu diễn các vecto qua vecto đơn vị: \(\overrightarrow u {\rm{ }} = {\rm{ }}\left( {a;{\rm{ }}b} \right) \Leftrightarrow \overrightarrow u = a\overrightarrow i + b\overrightarrow j \)

Lời giải chi tiết

a) Vẽ các vecto \(\overrightarrow {OA} = \overrightarrow a ,\overrightarrow {OB} = \overrightarrow b ,\overrightarrow {OC} = \overrightarrow c ,\overrightarrow {OD} = \overrightarrow d \)

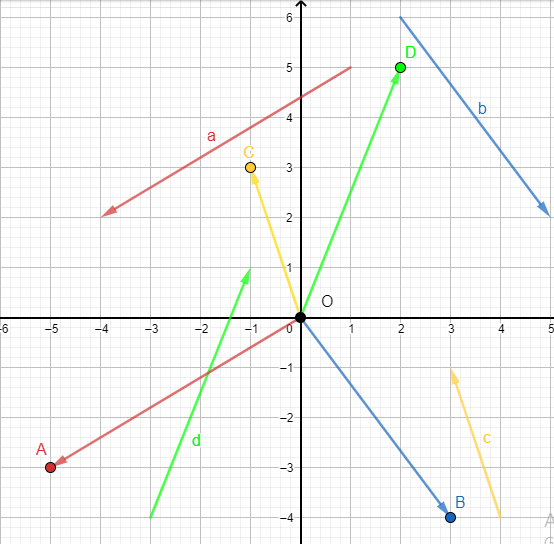
Dựa vào hình vẽ, ta thấy tọa độ của 4 điểm A, B, C, D là:

\(A\left( { - 5; - 3} \right),B\left( {3; - 4} \right),C\left( { - 1;3} \right),D\left( {2;5} \right)\)

Do đó \(\overrightarrow a = \overrightarrow {OA} = \left( { - 5; - 3} \right),\overrightarrow b = \overrightarrow {OB} = \left( {3; - 4} \right),\overrightarrow c = \overrightarrow {OC} = \left( { - 1;3} \right),\overrightarrow d = \overrightarrow {OD} = \left( {2;5} \right)\)

b) Vì \(\overrightarrow a = \overrightarrow {OA} = \left( { - 5; - 3} \right)\)nên \(\overrightarrow a = \left( { - 5} \right)\overrightarrow i + \left( { - 3} \right)\overrightarrow j = - 5\overrightarrow i - 3\overrightarrow j \)

Vì \(\overrightarrow b = \overrightarrow {OB} = \left( {3; - 4} \right)\) nên \(\overrightarrow b = 3\overrightarrow i + \left( { - 4} \right)\overrightarrow j = 3\overrightarrow i - 4\overrightarrow j \)

Vì \(\overrightarrow c = \overrightarrow {OC} = \left( { - 1;3} \right)\) nên \(\overrightarrow c = \left( { - 1} \right)\overrightarrow i + \left( 3 \right)\overrightarrow j = - \overrightarrow i + 3\overrightarrow j \)

Vì \(\overrightarrow d = \overrightarrow {OD} = \left( {2;5} \right)\) nên \(\overrightarrow d = 2\overrightarrow i + 5\overrightarrow j \)

Bình luận

Chia sẻ

Bình chọn:

4.1 trên 8 phiếu

Bài tiếp theo

Giải bài 2 trang 65 SGK Toán 10 tập 2 – Cánh diều
Tìm tọa độ của các vecto sau:
Giải bài 3 trang 65 SGK Toán 10 tập 2 – Cánh diều
Tìm các cặp số thực a và b sao cho mỗi cặp vecto sau bằng nhau:
Giải bài 4 trang 66 SGK Toán 10 tập 2 – Cánh diều
Trong mặt phẳng toạ độ Oxy, cho ba điểm A(2;3), B(-1; 1), C(3;- 1).
Giải bài 5 trang 66 SGK Toán 10 tập 2 – Cánh diều
Trong mặt phẳng toạ độ Oxy, cho điểm M(-1; 3). a) Tìm toạ độ điểm A đối xứng với điểm M qua gốc O. b) Tìm toạ độ điểm B đối xứng với điểm M qua trục Ox. c) Tìm toạ độ điểm C đối xứng với điểm M qua trục Oy.
Giải bài 6 trang 66 SGK Toán 10 tập 2 – Cánh diều
Trong mặt phẳng toạ độ Oxy, cho ba điểm không thẳng hàng A(- 3 ; 1), B(-1; 3), I(4;2). Tìm toạ độ của hai điểm C, D sao cho tứ giác ABCD là hình bình hành nhận I làm tâm đối xứng.
Giải bài 7 trang 66 SGK Toán 10 tập 2 – Cánh diều
Trong mặt phẳng toạ độ Oxy, cho tam giác ABC. Các điểm M(1;- 2), N(4;- 1) và P(6 ; 2) lần lượt là trung điểm của các cạnh BC, CA, AB. Tìm toạ độ của các điểm A, B, C.
Giải mục III trang 64 SGK Toán 10 tập 2 - Cánh diều
Trong mặt phẳng tọa độ Oxy, cho các điểm: A(1; 3) B(5; -1) C(2; -2) D(-2; 2)
Giải mục II trang 61, 62, 63 SGK Toán 10 tập 2 - Cánh diều
Cho điểm M trong mặt phẳng toạ độ Oxy. a) Vē vecto OM b) Nêu cách xác định toạ độ của điểm M.
Giải mục I trang 60 SGK Toán 10 tập 2 - Cánh diều
Trong mặt phẳng toạ độ Oxy (Hình 2), hãy: a) Tìm hoành độ và tung độ của điểm A. b) Nêu cách xác định toạ độ của điểm M tuỳ ý.