Môn Toán - Lớp 12
Môn Toán - Lớp 12
 40 bài tập trắc nghiệm giá trị lớn nhất, giá trị nhỏ nhất của hàm số mức độ vận dụng, vận dụng cao
40 bài tập trắc nghiệm giá trị lớn nhất, giá trị nhỏ nhất của hàm số mức độ vận dụng, vận dụng cao
Câu hỏi
Giá trị nhỏ nhất của hàm số \(f\left( x \right) = \frac{{{x^2} - x + 1}}{{x - 1}}\) trên khoảng \(\left( {1; + \infty } \right)\) là
- A \(\mathop {\min }\limits_{\left( {1; + \infty } \right)} y = 3.\)
- B \(\mathop {\min }\limits_{\left( {1; + \infty } \right)} y = - 1.\)
- C \(\mathop {\min }\limits_{\left( {1; + \infty } \right)} y = 5.\)
- D \(\mathop {\min }\limits_{\left( {1; + \infty } \right)} y = \frac{{ - 7}}{3}.\)
Phương pháp giải:
Sử dụng đạo hàm rồi lập bảng biến thiên.
Lời giải chi tiết:
Hàm số \(f\left( x \right) = \frac{{{x^2} - x + 1}}{{x - 1}}\) có đạo hàm \(f'\left( x \right) = \frac{{\left( {2x - 1} \right)\left( {x - 1} \right) - ({x^2} - x + 1)}}{{{{(x - 1)}^2}}} = \frac{{{x^2} - 2x}}{{{{\left( {x - 1} \right)}^2}}}\)
Ta có \(f'\left( x \right) = 0 \Leftrightarrow \frac{{{x^2} - 2x}}{{{{\left( {x - 1} \right)}^2}}} = 0 \Leftrightarrow \left[ \begin{array}{l}x = 0\\x = 2\end{array} \right.\)
\(f\left( x \right) \ge 0 \Leftrightarrow \left\{ \begin{array}{l}a > 0\\\Delta \le 0\end{array} \right.\)
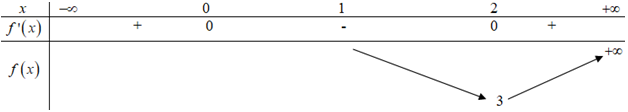
Bảng biến thiên:
Dựa vào bảng biến thiên ta có: hàm số đạt giá trị nhỏ nhất trên khoảng \(\left( {1; + \infty } \right)\) bằng 3.
Chọn A.