Câu hỏi
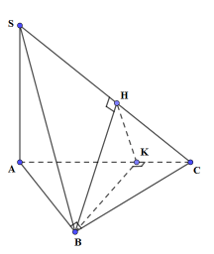
Cho hình chóp S.ABC có SA là đường cao và đáy là tam giác vuông tại B, BC = a. Hai mặt phẳng (SCA) và (SBC) hợp với nhau một góc \({60^0}\) và góc \(\widehat {BSC} = {45^0}\). Tính côsin của góc \(\alpha = \widehat {ASB}\).
- A \(\cos \alpha = \dfrac{{\sqrt 2 }}{2}\).
- B \(\cos \alpha = \dfrac{1}{{\sqrt 3 }}\).
- C \(\cos \alpha = \dfrac{{\sqrt 3 }}{2}\).
- D \(\cos \alpha = \sqrt {\dfrac{2}{5}} \).
Phương pháp giải:
Xác định góc giữa hai mặt phẳng \(\left( \alpha \right),\,\,\left( \beta \right)\):
- Tìm giao tuyến \(\Delta \) của \(\left( \alpha \right),\,\,\left( \beta \right)\).
- Xác định 1 mặt phẳng \(\left( \gamma \right) \bot \Delta \).
- Tìm các giao tuyến \(a = \left( \alpha \right) \cap \left( \gamma \right),b = \left( \beta \right) \cap \left( \gamma \right)\)
- Góc giữa hai mặt phẳng \(\left( \alpha \right),\,\,\left( \beta \right)\): \(\left( {\widehat {\left( \alpha \right);\left( \beta \right)}} \right) = \left( {\widehat {a;b}} \right)\) .
Lời giải chi tiết:
Kẻ \(BH \bot SC,BK \bot AC\).
Ta có: \(\left\{ \begin{array}{l}BK \bot AC\\BK \bot SA\end{array} \right. \Rightarrow BK \bot \left( {SAC} \right) \Rightarrow BK \bot SC\)
Mà \(BH \bot SC \Rightarrow SC \bot \left( {BHK} \right) \Rightarrow HK \bot SC\)
\(SC = \left( {SAC} \right) \cap \left( {SBC} \right) \Rightarrow \angle \left( {\left( {SAC} \right);\left( {SBC} \right)} \right) = \angle \left( {BH;HK} \right) = \angle BHK = {60^0}\)
Ta có: \(\left\{ \begin{array}{l}BC \bot AB\\BC \bot SA\end{array} \right. \Rightarrow BC \bot \left( {SAB} \right) \Rightarrow BC \bot SB\).
Mà \(\widehat {BSC} = {45^0} \Rightarrow \Delta SBC\) vuông cân tại B \( \Rightarrow \left\{ \begin{array}{l}SB = BC = a\\BH = \dfrac{{BC}}{{\sqrt 2 }} = \dfrac{a}{{\sqrt 2 }}\end{array} \right.\)
Đặt \(SA = x \Rightarrow A{B^2} = S{B^2} - S{A^2} = {a^2} - {x^2};\,\,A{C^2} = 2{a^2} - {x^2}\)
\(\Delta BHK\) vuông tại K, \(\widehat {BHK} = {60^0}\)
\( \Rightarrow HK = BH.\cos {60^0} = \dfrac{1}{2}BH = \dfrac{{a\sqrt 2 }}{4},\,\,BK = BH.\sin {60^0} = \dfrac{a}{{\sqrt 2 }}.\dfrac{{\sqrt 3 }}{2} = \dfrac{{a\sqrt 6 }}{4}\)
\(\Delta ABC\) vuông tại B, \(BK \bot AC \Rightarrow BK.AC = BC.AB\)
\(\begin{array}{l} \Leftrightarrow \dfrac{{a\sqrt 6 }}{4}.\sqrt {2{a^2} - {x^2}} = a.\sqrt {{a^2} - {x^2}} \\ \Leftrightarrow \dfrac{3}{8}\left( {2{a^2} - {x^2}} \right) = {a^2} - {x^2} \Leftrightarrow \dfrac{5}{8}{x^2} = \dfrac{{{a^2}}}{4} \Leftrightarrow x = a\sqrt {\dfrac{2}{5}} \end{array}\)
\( \Rightarrow \cos \alpha = \dfrac{{SA}}{{SB}} = \dfrac{{a\sqrt {\dfrac{2}{5}} }}{a} = \sqrt {\dfrac{2}{5}} \).
Chọn: D