Câu hỏi
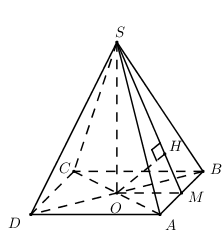
Cho hình chóp tứ giác đều \(S.ABCD\)đỉnh \(S\), khoảng cách từ \(C\) đến mặt phẳng\(\left( {SAB} \right)\) bằng \(6\). Gọi \(V\) là thể tích khối chóp \(S.ABCD\), tính giá trị nhỏ nhất của \(V\).
- A \(18\sqrt 3 \)
- B \(64\sqrt 3 \)
- C \(27\sqrt 3 \)
- D \(54\sqrt 3 \)
Phương pháp giải:
+) Đặt \(AB = x,\) tính \(SO\) theo \(x\) với \(O = AC \cap BD\).
+) \({V_{S.ABCD}} = \dfrac{1}{3}SO.{S_{ABCD}}\), sử dụng phương pháp hàm số tìm GTNN của hàm thể tích.
Lời giải chi tiết:
Gọi \(O = AC \cap BD \Rightarrow SO \bot \left( {ABCD} \right)\).
Ta có \(CO \cap \left( {SAB} \right) = A \Rightarrow \dfrac{{d\left( {C;\left( {SAB} \right)} \right)}}{{d\left( {O;\left( {SAB} \right)} \right)}} = \dfrac{{CA}}{{OA}} = 2\)
\( \Rightarrow d\left( {O;\left( {SAB} \right)} \right) = \dfrac{1}{2}d\left( {C;\left( {SAB} \right)} \right) = 3\).
Gọi \(M\) là trung điểm của \(AB\), trong \(\left( {SOM} \right)\) kẻ \(OH \bot SM\,\,\left( {H \in SM} \right)\) ta có:
\(\begin{array}{l}\left\{ \begin{array}{l}AB \bot OM\\AB \bot SO\,\,\left( {SO \bot \left( {ABCD} \right)} \right)\end{array} \right. \Rightarrow AB \bot \left( {SOM} \right) \Rightarrow AB \bot OH\\\left\{ \begin{array}{l}OH \bot SM\\OH \bot AB\end{array} \right. \Rightarrow OH \bot \left( {SAB} \right) \Rightarrow d\left( {O;\left( {SAB} \right)} \right) = OH = 3\end{array}\).
Đặt \(AB = x \Rightarrow OM = \dfrac{x}{2} > OH = 3 \Leftrightarrow x > 6\).
Áp dụng hệ thức lượng trong tam giác vuông ta có:
\(\dfrac{1}{{S{O^2}}} = \dfrac{1}{{O{H^2}}} - \dfrac{1}{{O{M^2}}} = \dfrac{1}{9} - \dfrac{4}{{{x^2}}} = \dfrac{{{x^2} - 36}}{{9{x^2}}} \Rightarrow SO = \dfrac{{3x}}{{\sqrt {{x^2} - 36} }}\,\,\left( {x > 6} \right)\).
Khi đó \({V_{S.ABCD}} = \dfrac{1}{3}SO.{S_{ABCD}} = \dfrac{1}{3}.\dfrac{{3x}}{{\sqrt {{x^2} - 36} }}.{x^2} = \dfrac{{{x^3}}}{{\sqrt {{x^2} - 36} }}\,\,\left( {x > 6} \right)\).
Xét hàm số \(f\left( x \right) = \dfrac{{{x^3}}}{{\sqrt {{x^2} - 36} }}\,\,\left( {x > 6} \right)\) ta có
\(\begin{array}{l}f'\left( x \right) = \dfrac{{3{x^2}\sqrt {{x^2} - 36} - {x^3}\dfrac{x}{{\sqrt {{x^2} - 36} }}}}{{{x^2} - 36}} = \dfrac{{3{x^2}\left( {{x^2} - 36} \right) - {x^4}}}{{\left( {{x^2} - 36} \right)\sqrt {{x^2} - 36} }} = \dfrac{{2{x^4} - 108{x^2}}}{{\left( {{x^2} - 4} \right)\sqrt {{x^2} - 4} }}\\f'\left( x \right) = 0 \Leftrightarrow 2{x^4} - 108{x^2} = 0 \Leftrightarrow 2{x^2}\left( {{x^2} - 54} \right) = 0 \Leftrightarrow x = 3\sqrt 6 \end{array}\).
\( \Rightarrow \mathop {\min }\limits_{\left( {6; + \infty } \right)} f\left( x \right) = f\left( {3\sqrt 6 } \right) = \dfrac{{{{\left( {3\sqrt 6 } \right)}^3}}}{{\sqrt {{{\left( {3\sqrt 6 } \right)}^2} - 36} }} = 54\sqrt 3 \)
Vậy \({V_{\min }} = 54\sqrt 3 \).
Chọn D.